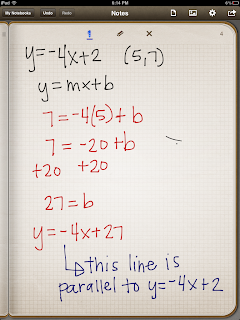December 14, 2011

Review of last week
Find a line that is perpendicular to another line

Remember: to find a perpendicular line the slope is the negative reciprocal
to find a parallel line the slope stays the same
always solve for b (the y intercept)

Writing Linear Equations

• You must have the slope and the y=intercept in order to write a linear equation
• to find the slope use the formula m = y2-y1/x2-x1
• to find the y-intercept use the formula b = y - mx

Example:

Encourage teachers to think like their students as they discuss the attributes of the different shapes.  What are rules you would use to sort these shapes?

#41

1. No straight edges vs straight edges

2.  No vertices vs vertices

3.  Line segments vs line segments

4.  How many curves/curved sides

5.  Parallel lines

6.  Arcs

7.  Concave vs convex

1. Concave vs Convex
2.  Vertices
3.  # of Arcs
4. Angles vs no angles

1.  # of sides
2.  Angles
3.  Concave vs. convex
4.  Regular polygons vs irregular
5.  # of peaks
6. Parallel vs nonparallel

How would you sort these shapes?

How would you sort these shapes?

Relationship of the interior angles of a triangle - they add up to 180 degrees

The total sum of the exterior angles of a triangle are 360 degrees

Sorting triangles

E = equilateral triangle
H = equilateral triangle
J = Right triangle, isosceles
A = right, scalene
F = Right, scalene
I = right, scalene
B = isosceles
C = isosceles
L = scalene
G = isosceles

Types of triangles : 

• scalene - all three sides are different lengths
• isosceles - two sides of the triangle are the same length
• equilateral - all three sides are equal in length
• acute - all three angles are acute angles
• obtuse - triangle has an obtuse angle
• right - triangle has a right angle

**Technically in geometry you don't classify triangles as acute or obtuse**

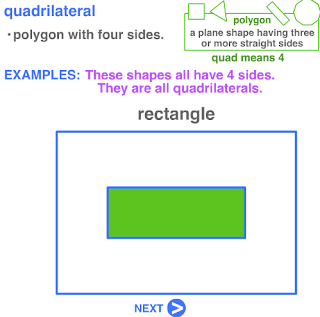
Quadrilaterals

properties of sides:one pair of parallel sides, if non-parallel sides are equal the shape is an isosceles trapezoid
properties of angles = can be 2 acute or 2 obtuse

2 sets of parallel lines
4 sides
angels: opposite angles are the same
properties of diagonals - nothing special here

Properties of sides - all sides are parallel and equal in lengs
Properties of angles: all right angles
diagonals: perpendicular, bisect, and congruent

Properties of sides: opposite sides are parallel, opposite sides are equal in length
Properties of angles: all angles are right angles
diagonals: are not perpendicular, they always bisect each other, diagonals will alway create identical triangles

properties of sides: all sides are equal in length
properties of angles: opposite angles are equal, 2 obtuse and 2 acute
diagonals: perpendicular and bisect

Number of sides - 2 = how many triangles
number of triangle x 180 = sum of interior angles

The more sides of a polygon the smaller the exterior angles.  All exterior angles add up to 360 degrees.

Homework

Read: Beckman 599-602

Measurement and Geometry

Measurement can be a difficult concept, especially for young students. Based on conversations from previous classes, we have discovered that students learn best when concepts are taught in a real world context. Teachers should focus on hands on activities to give students experience using measurement rather than just showing pictures and assigning worksheets. (Van de Walle, pg 369)

• ·         Describe everything you know about “standard units of measure."
•       Give examples of how you have used standard units of measure at home in the last week.
•       Give examples of how you have used non-standard units of measure at home in the last week.
•       Why is it important to have a standard system of measurement?

The three steps of Measurement

1. Make a Comparison
2. Concrete examples
3. Use of tools 

The Four Things we Measure

1. Time
2. Mass
   how many molecules (it is NOT weight).
   it is consistent
3. Distance or Length
4. Volume
   how much space it takes up

Geometry

• Geomoetry is a vocabulary course.
• Very important to know the definitions

1. Line
   never ending, infinite number of points
   must be straight
   no width
   
   
2. Angle
   made of two intersecting rays
   
   
   
3. Perpendicular Lines
   
   
   
4. Parallel Lines
   
   
5. Congruent
   
   
6. Degree
   
   
   
7. Postulate
   Things that can just be assumed based on general reasoning
   
8. Theorem
   must be proven
   A rule that can be used once it it proven
   
   

Parallel LinesThe slope will be the same for the parallel lines, the y intercept will be different

Perpendicular lines

The slopes are the negative reciprocal of each other.

Examples:

Transversal 

 - in class we talked about transversal being any line that intersects any set of parallel lines.  The transversal line does not have to be perpendicular.

Practice Drawing using verbal clues:

Supplementary

• two angles that add up to 180
• "supplementary makes a sunrise"

Complementary

• two angles that add up to 90
• "Compliments cause love, and love starts with an L"

Triangles:

Finding how many angles are in a triangle

This activity proves that there are 180 degrees in a triangle.

Vertical Angles

Interior Angles

Exterior Angle